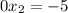Answer:
a) k=1, h=1, the unique solution of the system is
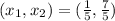
b) If k=6 and h=8 the system has infinite solutions.
c)If k=6 and h=3 the system has no solutions.
Explanation:
The augmented matrix of the system is
![\left[\begin{array}{ccc}1&3&4\\2&k&h\end{array}\right]](https://img.qammunity.org/2020/formulas/mathematics/college/nd3ytzq6be9qkvpjwxakvb3lwlkt2ayt6z.png) . If two times the row 1 is subtracted to row 2 we get the following matrix
. If two times the row 1 is subtracted to row 2 we get the following matrix
![\left[\begin{array}{ccc}1&3&4\\0&k-6&h-8\end{array}\right]](https://img.qammunity.org/2020/formulas/mathematics/college/q2nm4d52izbbrwhtallih8v7i2azm4cp2w.png) .
.
Then
a) If k=1 and h=1, the unique solution of the system is
 and solviong for
and solviong for
 ,
,
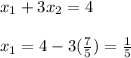
Then the solution is
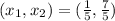
b) If k=6 and h=8 the system has infinite solutions because the echelon form of the matrix has a free variable.
c)If k=6 and h=3, the system has no solutions because the last equation of the system of the echelon form of the matrix is