Answer:
Given :Cost function :
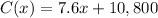
To Find : Find the average cost per item when the required number of items is produced
Solution:
a) 200 items
Cost function :
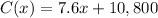
Substitute x = 200
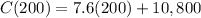

So, Total cost of producing 200 items is 12320
Now Average cost per item =

Average cost per item=
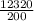
Average cost per item=61.6
So, the average cost per item when 200 items are produced is 61.6 .
b) 2000 items
Cost function :
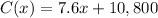
Substitute x = 2000
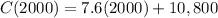
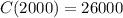
So, Total cost of producing 2000 items is 26000
Now Average cost per item =

Average cost per item=
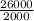
Average cost per item=13
So, the average cost per item when 2000 items are produced is 13.
c) 5000 items
Cost function :
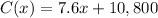
Substitute x = 5000

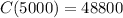
So, Total cost of producing 5000 items is 48800
Now Average cost per item =

Average cost per item=
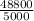
Average cost per item=9.76
So, the average cost per item when 5000 items are produced is 9.76