Question

1 . Perpetuities Perpetuities are also called annuities with an extended or unlimited life. Based on your understanding of perpetuities, answer the following questions. Which of the following are characteristics of a perpetuity? Check all that apply. The value of a perpetuity is equal to the sum of the present value of its expected future cash flows. A perpetuity is a stream of unequal cash flows. The current value of a perpetuity is based more on the discounted value of its nearer (in time) cash flows and less by the discounted value of its more distant (in the future) cash flows. A perpetuity is a stream of regularly timed, equal cash flows that continues forever. A local bank’s advertising reads: "Give us $50,000 today, and we’ll pay you $800 every year forever." If you plan to live forever, what annual interest rate will you earn on your deposit? 1.60% 2.24% 1.28% 1.44% Oops! When you went in to make your deposit, the bank representative said the amount of required deposit reported in the advertisement was incorrect and should have read $75,000. This revision, which will the interest rate earned on your deposited funds, will adjust your earned interest rate to .

Answer 1

(A) A perpetuity is a stream of regularly timed, equal cash flows that continues forever

(B) The value of a perpetuity is equal to the sum of the present value of its expected future cash flows

the bank offers 1.6%

in the alternative scenario it offers 1.067%

Step-by-step explanation:

(A) A perpetuity is a stream of regularly timed, equal cash flows that continues forever

The perpetuity is an annuity in which time tends to infinity, to be qualified as an annuity the cash payment must be regular.

(B) The value of a perpetuity is equal to the sum of the present value of its expected future cash flows

As state above the perpetuinty is an annuity, the annuities return the present value of the expcted future cash flow.

Given the annuity formula

`C * (1-(1+r)^(-time) )/(rate) = PV\\`

if times tends to infinity then the expression:

`\lim_(n \to \infty) (1+r)^(-n) = 1`

Nexti n the annuity formula we got:

`C * (1-1 )/(rate)= PV\\`

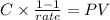
So we end up with C / rate = PV

which s the perpetuity formula

800/50000 = 0.016 = 1.6%

800/75000 = 0.0106667 = 1.067%