Answer:
9b) -8 = y
9a) 72° = m∠ABC
Explanation:
Since you have an angle trisector, in this case, m∠CBE ≅ m∠DBA, therefore you set x equal to 8, plus, according to Morley's Trisector Theorem, all three angles form an equilateral triangle, so m∠BOC also has to equal 24°:
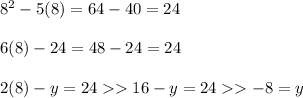
Then, m∠ABC comes from multiplying 3 by 24 [three twenty-four's], which results in 72°.
I am joyous to assist you anytime.