Answer:
The answer is D. 1/5
Explanation:
Let´s write equation for each of the senteneces in the question:
- Alice saved the same fraction for her take-home pay (THP) each month => Savings_month = X*THP, where "X" is the fraction of the THP she saves.
- She saved 3 times the amount of money she didn´t save by the end of 12 months => Savings_12months = 12*X*THP = 3*Y*THP, where "Y" is the fraction of the money she did not save
- We can also conclude that the THP is the sum of her savings and what she did not save => THP = X*THP + Y*THP
We have 2 equations:
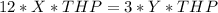
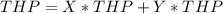
THP is on both sides on both equations, that means we can divide the equation on both sides by THP to get rid of it and have easier to read equations:
- 12X = 3Y
- 1 = X + Y
From equation 1, solve for Y and we get the following:
Substitute Y in equation 2:
Solve for X:
We know that X is the fraction of THP Alice is saving, therefore the answer is D.