Answer: 1.47
Step-by-step explanation:
The combined gas equation when pressure is constant:
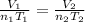
where,
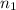 =original number of moles of air in the balloon = ?
=original number of moles of air in the balloon = ?
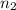 = number of moles of air in the heated balloon = ?
= number of moles of air in the heated balloon = ?
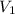 = initial volume of gas =
= initial volume of gas =
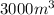
 = final volume of gas =
= final volume of gas =

 = initial temperature of gas =
= initial temperature of gas =

 = final temperature of gas =
= final temperature of gas =
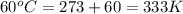
Now put all the given values in the above equation, we get the final pressure of gas.
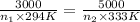
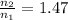
Therefore, the ratio of the number of moles of air in the heated balloon to the original number of moles of air in the balloon is 1.47