Question

Announcers at a certain radio station say that they are at "95 FM on your dial," meaning that they transmit at a frequency of 95 MHz (95 megahertz, or 95 million cycles per second). What is the wavelength of the radio waves from this station

Answer 1

`\lambda=3.15\ m`

Step-by-step explanation:

Frequency of the FM,
`f=95\ MHz=95* 10^6\ Hz`

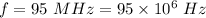
We need to find the wavelength of the radio waves from this station. The relationship between the frequency and the wavelength is given by :

`\lambda=(c)/(f)`

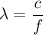
c is the speed of light

`\lambda=(3* 10^8\ m/s)/(95* 10^6\ Hz)`

`\lambda=3.15\ m`

So, the wavelength of the radio waves from this station is 3.15 meters. Hence, this is the required solution.