Answer:
245.86 lb of salt
Step-by-step explanation:
Let y(t) be the amount of salt in the tank at instant t. Then y'(t) represents the rate of change of the amount of salt in the tank at instant t.
The water entering the tank contains 14 lb of salt per gallon and enters at a speed of 4 gal/min, so every minute 14*4=56 lb of salt are entering the tank the first 8 min.
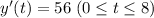
After 8 minutes, as the volume of tank keeps 160 gal., the concentration of salt is
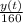 .
.
Since the water is now leaving the tank at 6 gal/min, the salt is leaving the tank at a speed of
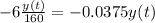 .
.
Notice that the speed is negative because the salt is leaving the tank, so the amount of salt is decreasing.
Now we have the following ordinary differential equation
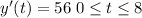
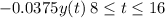
Solving this differential equation we get a piecewise function defined as follow
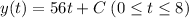
where C is a constant.
Since the tank had no salt at the beginning of the process. Y(0)=0, so

For
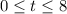 we have
we have
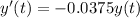
and solving this equation we get

where C is a constant that can be found with the initial condition y(8) = 56*8 = 448
and our solution is
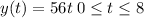
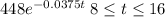
To know what the amount of salt is at the end of the process, we must evaluate y(16), which is
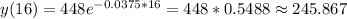
So, at the end of the process the tank contains 245.867 lb of salt.