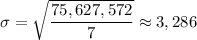Question

Suppose the following data set represents the starting salaries for the population of teachers from seven states: $41,027, $35,901, $31,159, $33,664, $35,166,$39,338, $32,691

A) calculate the mean of the data set
B) calculate the 5 number summary
C) calculate the standard deviation of this data set

Answer 1

See explanation

Explanation:

Arrange the salaries $41,027, $35,901, $31,159, $33,664, $35,166, $39,338, $32,691 in ascending order:

$31,159, $32,691, $33,664, $35,166, $35,901, $39,338, $41,027

A) The mean salary (to the nearest dollar) is

`(\$31,159+\$32,691+\$33,664+\$35,166+\$35,901+\$39,338+\$41,027)/(7)=\$35,564`

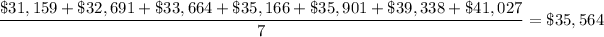
B) Minimum
`= \$31,159`

`Q_1=\$32,691`

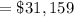
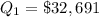
Median
`=\$35,166`

`Q_2=\$39,338`

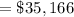
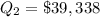
Maximum
`=\$41,027`

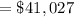
C) The standard deviation of this data set can be calculated using formula

`\sigma=\sqrt{\frac{\sum(x_i-\text{mean})^2}{n}}`

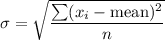
First, find
`(x_i-\text{mean})^2:`

`(31,159-35,564)^2=19,404,025\\ \\(32,691-35,564)^2=8,254,129\\ \\(33,664-35,564)^2=3,610,000\\ \\(35,166-35,564)^2=158,404\\ \\(35,901-35,564)^2=113,569\\ \\(39,338-35,564)^2=14,243,076\\ \\(41,027-35,564)^2=29,844,369`

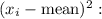
Now,

`\sigma =\sqrt{(75,627,572)/(7)}\approx 3,286`