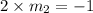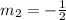Answer:
Slope of a line perpendicular to the line AB =
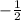
Slope of a line parallel to AB = 2
Explanation:
Let slope of a line =
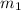
And slope of the other line is =
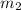
If these lines are parallel then

If these lines are perpendicular then
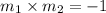
Slope of the line passing through two points (x, y) and (x', y') =
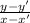
Therefore, slope of the line passing through A(3, 4) and B(5, 8) =
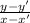
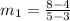
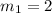
a). If a line having slope
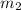 is parallel then
is parallel then

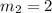
b). If a line having slope
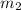 is perpendicular then
is perpendicular then