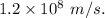Answer:
Velocity of the electron = v = 1.2\times 10^8\ m/s.
Step-by-step explanation:
Given,
- Mass of the electron =

- Charge on the electron =
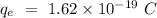
- Charge density of the ring =
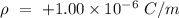
- Radius of the ring = R = 0.70 m
- Distance between the electron ant the center or the ring = x = 0.5 m
Now total charge on the ring =
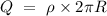
Potential energy due to the charged ring to the point on the x-axis is
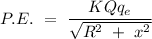
Let v be the velocity of the electron at the center of the ring.
Total kinetic energy of the electron =
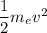
Now, From the conservation of energy,
the total potential energy of the electron at initially is converted to the total kinetic energy of the electron at the center of the ring,

Hence the velocity of the electron on the center of the charged ring is