Question

The amount of time it takes to recover physiologically from a certain kind of sudden noise is found to be normally distributed with a mean of 80 seconds and a standard deviation of 10 seconds. Using the 50%-34%-14% figures, approximately what percentage of scores (on time to recover) will be (a) above 100, (b) below 100, (c) above 90, (d) below 90, (e) above 80, (f) below 80, (g) above 70, (h) below 70, (i) above 60, and (j) below 60?

Answer 1

a) 2.5%

b) 97.5%

c) 16%

d) 84%

e) 50%

f) 50%

g) 84%

h) 16%

i) 97.5%

j) 2.5%

Explanation:

The empirical rule, or the rule of 50%-34%-14%, states that:

In a normally distributed stat with mean
`\mu` and standard deviation
`\sigma`...

a) 2.5% of the scores are going to be above
`\mu + 2\sigma`

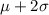
b) 13.5% of the scores are going to be above
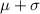`\mu + \sigma` and below
`\mu + 2\sigma`.

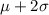c) 34% of the scores are going to be above
`\mu` and below
`\mu + \sigma`

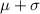
d) 34% of the scores are going to be above
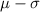`\mu - \sigma` and below
`\mu`

e) 13.5% of the scores are going to be above
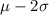`\mu - 2\sigma` and below
`\mu - \sigma`

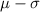
f) 2.5% of the scores are going to be below
`\mu - 2\sigma`

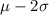
In this problem

We have that
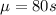`\mu = 80s` and
`\sigmma = 10s`

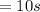
So:

(a) above 100, (b) below 100

`100 = \mu + 2\sigma = 80 + 2*10`

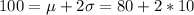
So 2.5% of the scores are going to be above 100, and the other 97.5% is going to be below 100

c) above 90, (d) below 90

`90 = \mu + \sigma = 80 + 10`

So 13.5% of the scores are going to be above 90 and below 100, and 2.5% of the scores are going to be above 100. So 13.5% + 2.5% = 16% of the scores are going to be above 90 and the other 84% is going to be below 90

(e) above 80, (f) below 80

80 is the mean, so approximately 50% percent of the scores are going to be above 80 and 50% are going to be below 70%.

(g) above 70, (h) below 70

`70 = \mu - \sigma = 80 - 10`

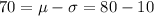
34% of the scores are going to be above 70 and below 80, and other 50% percent of the scores are going to be above 80. So in all, 84% of the scores are going to be above 70. The other 16% of the scores are going to be below 70.

(i) above 60, and (j) below 60

`60= \mu - 2\sigma = 80 - 2*10`

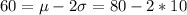
So 2.5% of the scores are going to be below 60, and the other 97.5% is going to be above 100