Question

determine algebraically if the following functions are even,odd,or neither.if even state the symmetry

Answer 1

`{ \qquad\qquad\huge\underline{{\sf Answer}}}`

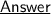
The given function is :

`\qquad \sf  \dashrightarrow \: f(x) = 3 {x}^(3) - x`

`{\qquad \sf  \dashrightarrow \: f (-x) = 3 (-x)³ - (-x)}`

`{\qquad \sf  \dashrightarrow \: f (-x) = - 3x³ + x}`

`{\qquad \sf  \dashrightarrow \: f (-x) = -( 3x³ - x)}`

`{\qquad \sf  \dashrightarrow \: f(-x) = - f(x)}`

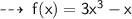
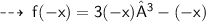
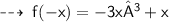
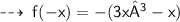
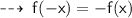
Hence, it's an odd function.

Also, the polynomial has odd powers, there is different value of y for each and every value of x, even for numbers and their additive inverse. and there will be symmetry in it about (0 , -3)

Therefore it's an odd function.