Answer: Our required probability is 0.745.
Explanation:
Since we have given that
Probability of getting good risks people = 0.05
Probability of getting average risks people = 0.15
Probability of getting bad risks people = 0.30
Probability of getting good risk accident = 0.2
Probability of getting average risk accident = 0.5
Probability of getting bad risk accident = 0.3
So, Probability of people having accident in a year is given by
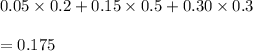
Probability of people either good or average risk given that he\she had no accident is given by

Hence, our required probability is 0.745.