Question

When a customer places an order at Ying Ying's bakery, there is an 8\%8%8, percent probability that the customer will report a food allergy. One day, 121212 customers place orders at Ying Ying's bakery.

Assuming that each of the 121212 customers is equally likely to report a food allergy, what is the probability that at least one customer will report a food allergy?

Answer 1

The sampling distribution of (p^c-p^a) will not be normal, because we expect fewer than 10 children to redeem the coupon.

Explanation:

khan

Answer 2

Answer: 0.6323

Explanation:

Given : The proportion of customer will report a food allergy : p=0.08

One day, 121 customers place orders at Ying Ying's bakery.

If we assume that each of the 12 customers is equally likely to report a food allergy , then we can use Binomial distribution.

Formula for Binomial probability distribution:

`P(X=x)=^nC_xp^x(1-p)^(n-x)`

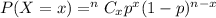
, where P(x)= probability of getting success in x trials, n= sample size , p= probability of getting success in each event.

As per given , we have n= 12

p=0.08

Let x denotes the customer will report a food allergy.

Then, the probability that at least one customer will report a food allergy will be :

`P(x\geq1)=1-P(x<1)\\\\=1-P(x=0)\\\\=1-^(12)C_0(0.08)^0(1-0.08)^(12-0)\\\\=1-(1)(0.92)^(12)\ \ [\because\ ^nC_0=1]\\\\\approx1-0.3677=0.6323`

Hence, the required probability = 0.6323