Answer:
Option 3 - f(x)= 4x,
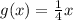
Explanation:
To find : Which two functions are inverses of each other?
Solution :
Two functions are inverse if

Now, we find one by one
1) f(x)= x, g(x) = -x
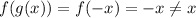
Not true.
2) f(x)= 2x,

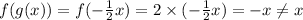
Not true.
3) f(x)= 4x,
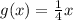
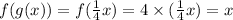
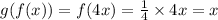
i.e.
 is true.
is true.
So, These two functions are inverse of each other.
4) f(x)= -8x,
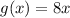

Not true.
Therefore, Option 3 is correct.