Answer:
Q = 4 Q₀
Step-by-step explanation:
This is an exercise on capacitors, where the capacitance is
C =
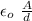
if we apply the given conditions
C = \epsilon_{o} \ \frac{2A}{0.5d}
C = 4 \epsilon_{o} \ \frac{A}{d}
let's call the capacitance Co with the initial values
C₀ = \epsilon_{o} \ \frac{A}{d}
C = 4 C₀
The charge on each plate of a capacitor is
Q = C ΔV
If the potential difference is maintained, the new charge is
Q = 4 C₀ ΔV
let's call
Q₀ = C₀ ΔV
we substitute
Q = 4 Q₀