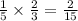Explanation:
Let's change the denominator of
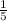
to 15 as it is the lowest common multiple of both denominators, 5 and 3.
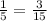
Now, think of it this way, there are 15 people cycling and
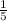
of them cycle to work.
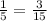
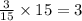
Out of these 15 cyclists, 3 of them go to work. As

of these remainders cycle via mountain bikes, we can find how many people ride mountain bikes to work.
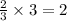
As there was a total of 15 people at the start and 2 who rode mountain bikes to work, the fraction of the population who rides mountain bikes to work will be
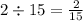
Short answer: