Question

The editor of a textbook publishing company is deciding whether to publish a proposed textbook. Information on previous textbooks published show that 10 % are huge​ successes, 20 % are modest​ successes, 50 % break​ even, and 20 % are losers. Before a decision is​ made, the book will be reviewed. In the​ past, 99 % of the huge successes received favorable​ reviews, 60 % of the moderate successes received favorable​ reviews, 40 % of the​ break-even books received favorable​ reviews, and 20 % of the losers received favorable reviews. If the textbook receives a favorable​ review, what is the probability that it will be huge​ success?

Answer 1

If the textbook receives a favorable review, there is a 21.57% probability that it will be a huge success.

Explanation:

We have the following probabilities:

-A 10% probability that the textbook is a huge success.

-A 20% probability that the textbook is a modest success.

-A 50% probability that the textbook breaks even

-A 20% probability that the textbook is a loser

-If the book is a huge success, there is a 99% probability that it receives favorable reviews.

-If the book is a moderate success, there is a 60% probability that it receives favorable reviews.

-If the book breaks even, there is a 40% probability that it receives favorable reviews.

-If the book is a loser, there is a 20% probability that it receives favorable reviews.

If the textbook receives a favorable​ review, what is the probability that it will be huge​ success?

This can be formulated as the following problem:

What is the probability of B happening, knowing that A has happened.

It can be calculated by the following formula

`P = (P(B).P(A/B))/(P(A))`

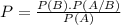
Where P(B) is the probability of B happening, P(A/B) is the probability of A happening knowing that B happened and P(A) is the probability of A happening.

So, for this problem.

What is the probability that the book is a huge success, given that it received favorable reviews?

P(B) is the probability that the book is a huge success. So:

`P(B) = 0.1`

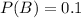
P(A/B) is the probability that the book receives favorable reviews when it is a huge success. So:

`P(A/B) = 0.99`

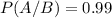
P(A) is the probability that the book receives favorable reviews:

`P(A) = P_(1) + P_(2) + P_(3) + P_(4)`

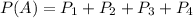
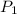`P_(1)` is the probability that a book that is a huge success is chosen and receives favorable reviews. So:

`P_(1) = 0.1*0.99 = 0.099`

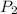`P_(2)` is the probability that a book that is a moderate success is chosen and receives favorable reviews. So:

`P_(2) = 0.2*0.6 = 0.12`

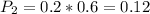
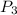`P_(3)` is the probability that a book that breaks even is chosen and receives favorable reviews. So:

`P_(3) = 0.5*0.4 = 0.20`

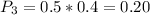
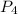`P_(4)` is the probability that a book that is a loser is chosen and receives favorable reviews. So:

`P_(4) = 0.20*0.20 = 0.04`

So

`P(A) = P_(1) + P_(2) + P_(3) + P_(4) = 0.099 + 0.12 + 0.20 + 0.04 = 0.459`

If the textbook receives a favorable​ review, what is the probability that it will be huge​ success?

`P = (P(B).P(A/B))/(P(A)) = (0.1*0.99)/(0.459) = 0.2157`

If the textbook receives a favorable review, there is a 21.57% probability that it will be a huge success.