Question

At elevated temperatures, dinitrogen pentoxide decomposes to nitrogen dioxide and oxygen: 2N2O5(g) → 4NO2 (g) + O2 (g) When the rate of formation of NO2 is 5.5 ⋅ 10-4 M/s, the rate of decomposition of N2O5 is ________ M/s. At elevated temperatures, dinitrogen pentoxide decomposes to nitrogen dioxide and oxygen: 2N2O5(g) 4NO2 (g) + O2 (g) When the rate of formation of NO2 is 5.5 10-4 M/s, the rate of decomposition of N2O5 is ________ M/s. 2.8 ⋅ 10-4 1.4 ⋅ 10-4 5.5 ⋅ 10-4 10.1 ⋅ 10-4 2.2 ⋅ 10-3

Answer 1

Answer : The rate of decomposition of
`N_2O_5` is
`2.8* 10^(-4)M/s`

Explanation : Given,

Rate of formation of
`NO_2` =
`5.5* 10^(-4)M/s`

The given rate of reaction is,

`2N_2O_5(g)\rightarrow 4NO_2(g)+O_2(g)`

The expression for rate of reaction :

`\text{Rate of decomposition of }N_2O_5=-(1)/(2)* (d[N_2O_5])/(dt)`

`\text{Rate of formation of }NO_2=+(1)/(4)(d[NO_2])/(dt)`

`\text{Rate of formation of }O_2=+(d[O_2])/(dt)`

Now we have to calculate the rate of decomposition of
`N_2O_5`.

As we know that,

`\text{Rate of reaction}=-(1)/(2)* (d[N_2O_5])/(dt)=+(1)/(4)(d[NO_2])/(dt)=+(d[O_2])/(dt)`

So,

`-(1)/(2)* (d[N_2O_5])/(dt)=+(1)/(4)(d[NO_2])/(dt)`

`-(d[N_2O_5])/(dt)=+(2)/(4)(d[NO_2])/(dt)`

`-(d[N_2O_5])/(dt)=(2)/(4)* (5.5* 10^(-4)M/s)`

`-(d[N_2O_5])/(dt)=2.8* 10^(-4)M/s`

Thus, the rate of decomposition of
`N_2O_5` is
`2.8* 10^(-4)M/s`

Answer 2

The rate of descomposition of N₂O₅ is: 2,8 M/s

Step-by-step explanation:

The global reaction is:

2 N₂O₅ (g) → 4 NO₂ (g) + O₂ (g)

The problem says that rate of formation of NO₂ = 5,5x10⁻⁴ M/s where M is molarity -
`(mol)/(L)`-

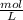The rate of descomposition of N₂O₅ is:

5,5x10⁻⁴
`(molNO_(2) )/(L.s)` ×
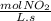`(2 mol N_(2)O_(5))/(4 mol NO_(2) )` = 2,8 M/s

I hope it helps!