Answer:
8 cm and 15 cm.
Explanation:
Let x and y represent length of each leg of right triangle.
We have been given that the perimeter of a right triangle is 40 cm, and its hypotenuse measures 17 cm.
We can represent this information in a system of equations as:
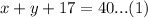
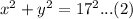
From equation (1), we will get:
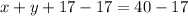
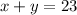
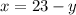
Substitute this value in equation (2):
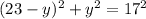
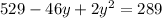
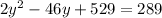
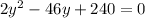

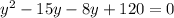
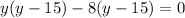

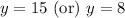
Therefore, the length of the legs of the hypotenuse would be 8 cm and 15 feet.