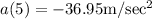Question

The acceleration of a particle traveling along the x axis is given by a(t) = −bt, where b = 7.39 m/s3. In addition, we know that at t = 0, the particle is at the position x0 = 5.00 m and has a velocity of v0 = 10.0 m/s. At the time t = 5.00 s, determine the following for this particle. (Indicate the direction with the sign of your answer.)

Answer 1

Acceleration of the particle=
`-36.95 \mathrm{m} / \mathrm{sec}^(2)`

Velocity of the particle=
`-82.375 \mathrm{m} / \mathrm{sec}`

position of the particle=
`98.985 \mathrm{m} / \mathrm{sec}`

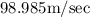
Step-by-step explanation:

Given:

`b=7.39 \mathrm{m} / \mathrm{s}^(3)`

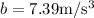
t = 0

`X_(0)=5.00 \mathrm{m}`

`V_(0)=10.0 \mathrm{m} / \mathrm{s}`

`X_(0)=5.00 \mathrm{m}`

`V_(0)=10.0 \mathrm{m} / \mathrm{s}`

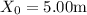
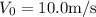
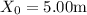
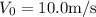
To find:

The acceleration of particle at t= 5,00 s

Velocity of the particle at t= 5,00 s

Postion of the particle at t= 5,00 s

Solution:

Finding the value of a:

`v(t)=\int a(t) . b(t)=\left((-b t^(2))/(2)+a\right) m / s e c`

`\left((-7.39 t^(2))/(2)+a\right)_(0)=10`

`\left((-b t^(2)+2 a)/(2)\right)_(0)=10`

`\left(-b t^(2)+2 a\right)_(0)=2(10)`

`\left(-b t^(2)+2 a\right)_(0)=20`

`\left(-b(0)^(2)+2 a\right)=2(10)`

`2 a=20`

`a=10`

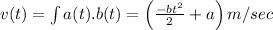
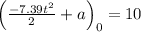
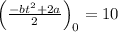
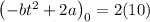
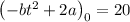
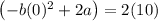
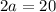
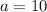
Finding the value of c

`x(t)=\int v(t) \cdot d(t)=\left((-b t^(3))/(6)+a t+c\right) m / s e c`

`\left((-b t^(3))/(6)+a t+c\right)_(0)=5`

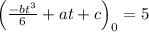
Substituting t=0

`\left((-b(0)^(3))/(6)+a(0)+c\right)=5`

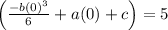
c=5

Finding the velocity of of particle at t=5

`v(t)=\int a(t) . b(t)=\left((-b t^(2))/(2)+a\right) m / s e c`

`v(5)=\left((-(7.39)(5)^(2))/(2)+10\right)`

`v(5)=\left((-(7.39)(25))/(2)+10\right)`

`v(5)=\left((-(184.75))/(2)+10\right)`

`v(5)=\left((-(184.75))/(2)+10\right)`

`v(5)=(-92.375+10)`

`v(5)=(-82.375)`

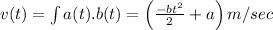
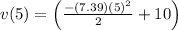
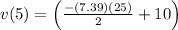
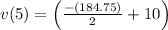
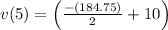
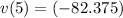
Position of the particle at x=5

`x(t)=\int v(t) \cdot d(t)=\left((-b t^(3))/(6)+a t+c\right) m`

`x(5)=\left((-(7.39)(5)^(3))/(6)+(10)(5)+5\right)`

`x(5)=\left((-(7.39)(25))/(6)+(50)+5\right)`

`x(5)=\left((-(923.75))/(6)+(50)+5\right)`

`x(5)=(-153.958+(50)+5)`

`x(5)=(-153.958+(55)`

`x(5)=98.985 \mathrm{m}`

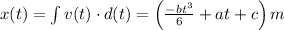
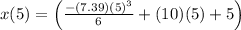
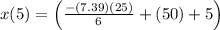
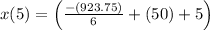
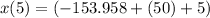
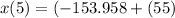
The acceleration of the particle t=5

`a(t)=-b t`

`a(5)=-(7.39)(5)`

`a(5)=-36.95 \mathrm{m} / \mathrm{sec}^(2)`