Question

Simplify the expression. Write your answer as a complex number. 2 times the square root of 25 plus the square root of negative 16 10 + 4i 10 − 4i 10 + 8i 10 − 8i

Answer 1

10 + 4i

Explanation:

`\begin{array}{rcl}2√(25) + √(-16) & = & 2 * 5 + √((-1)(16))\\& = & \mathbf{10 + 4i}\\\end{array}`

Answer 2

A.
`10+4i`

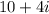
Explanation:

We are asked to simplify the given expression
`2* √(25)+√(-16)`.

We will use imaginary unit 'i' to solve our given problem.

Write 25 as square of 5:

`2* √(5^2)+√(-16)`

`2* 5+√(-16)`

`10+√(-16)`

`10+√(-1* 16)`

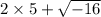
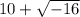
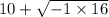
Substitute
`i^2=-1` in the expression:

`10+√(i^2* 16)`

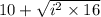
Write 16 as square of 4:

`10+√(i^2* 4^2)`

`10+4i`

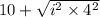
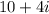
Therefore, the simplified form of our given expression would be
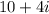`10+4i`.