Answer:
120 mi/h
Step-by-step explanation:
Given:
 = initial speed from where you start = 0 mi/h
= initial speed from where you start = 0 mi/h
 = acceleration for your drive =
= acceleration for your drive =
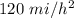
 = total distance between Austin and Houston = 180 mi
= total distance between Austin and Houston = 180 mi
 = total time remaining for the journey = 2 h
= total time remaining for the journey = 2 h
Assume:
 = time for which you drive with constant acceleration
= time for which you drive with constant acceleration
 = the remaining time for which you drive for the rest distance =
= the remaining time for which you drive for the rest distance =

 = maximum speed reached by you in the entire journey
= maximum speed reached by you in the entire journey
When you start from rest and accelerate with constant acceleration for time t, you reaches your maximum speed at the last instant time of this interval. Let us first find out this speed.
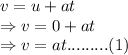
After you reach your maximum speed, you travel for the rest of the journey with this much speed. This means the sum of distances traveled during the constant acceleration and the constant speed is equal to the total distance between Austin and Houston.
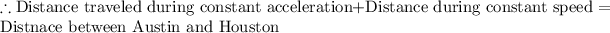

But t = 3 h is greater than the maximum allowed time.
So, t = 1 h is correct.
This means the drive must have a constant acceleration of
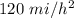 for 1 h.
for 1 h.
Now, on putting the value of time in equation (1), we have
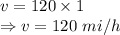
Hence, the speed you will be going during the trip is 120 mi/h.