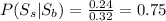Answer: P = 0.75
Explanation:
Hi!
The sample space of this problems is the set of all the possible sales. It is divided in the disjoint sets:
We have also the set of sales of boat accesories
 , the colored one in the image.
, the colored one in the image.
We are given the data:
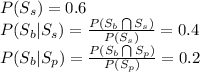
From these relations you can compute the probabilities of the intersections colored in the image:
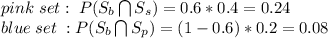
You are asked about the conditional probability:
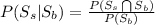
To calculate this, you need
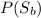 . In the image you can see that the set
. In the image you can see that the set
 is the union of the two disjoint pink and blue sets. Then:
is the union of the two disjoint pink and blue sets. Then:
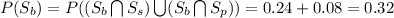
Finally: