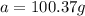Answer:
Part a)

Part b)
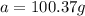
Step-by-step explanation:
Part a)
During the launch
speed will increase from rest to final speed of 1.60 m/s
the distance moved by it is given as
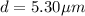
now we have
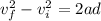
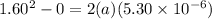
so we have
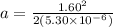
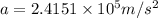
now in terms of g it is given as

Part b)
During speed reduction
we know that final speed will be zero by air in distance 1.30 mm
so we have
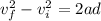
![0 - 1.60^2 = 2(a)(1.30 * 10^(-3))/tex]</p><p>[tex]a = 984.6 m/s^2](https://img.qammunity.org/2020/formulas/physics/high-school/erpfkmd846ggy1o9n6tdeb5yshwnlkfrxk.png)
Now in terms of g it is