The midpoint of RS is M(1, 2). One endpoint is R(-6, 6). Find the coordinates of endpoint S.

Question

asked Nov 22, 2020 123k views

1 Answer

Sky Notify · Answer 1 · 2020-11-28T19:56:50+0000

Answer:

The coordinates of point S are (8,-2)

Explanation:

we know that

The formula to calculate the midpoint between two points is equal to

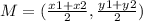
M=((x1+x2)/(2),(y1+y2)/(2))

Let

Point S(x2,y2)

substitute the given values

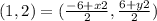
(1,2)=((-6+x2)/(2),(6+y2)/(2))

Solve for x2

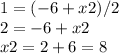
$1=(-6+x2)/2\\2=-6+x2\\x2=2+6=8$

Solve for y2

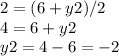
$2=(6+y2)/2\\4=6+y2\\y2=4-6=-2$

therefore

The coordinates of point S are (8,-2)