Question

A football quarterback is moving straight backward at a speed of 1.91 m/s when he throws a pass to a player 16.1 m straight downfield. The ball is thrown at an angle of 37.3° relative to the ground and is caught at the same height as it is released. What is the initial velocity of the ball relative to the quarterback? (Assume downfield to be in the +x-direction. Enter the magnitude in m/s and the direction in degrees counterclockwise from the +x-axis.)

Answer 1

`V_(b/p)=14.48 m/s` at an angle of 31.24°

Step-by-step explanation:

We know that
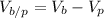`V_(b/p) = V_(b)-V_(p)` so we need the velocity of the ball relative to ground.

The equations for the ball in X and Y are these ones:

`X_(b)=V_(b)*cos(37.3)*t`

`Y_(b)=V_(b)*sin(37.3)*t-(g*t^(2))/(2)`

From X-axis equation:

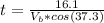`t=(16.1)/(V_(b)*cos(37.3))` Replacing this value in the Y-axis equation we can find Vb:

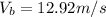`V_(b)=12.92m/s` And this is the magnitude relative to ground. The components are:

`V_(b)=[12.92*cos(37.3),12.92*sin(37.3)]=[10.28,7.83]m/s`

Now we calculate the velocity of the ball relative to the player as:

`V_(b/p) = V_(b)-V_(p)=[12.19,7.83]m/s` If we now calculate the magnitude and the angle of this vector, we get the answer to the problem:

`V_(b/p) = (14.48 < 31.24°)m/s`