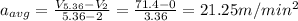Answer:
a)
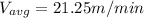
b)
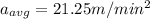
c)
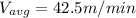
d)
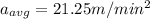
Step-by-step explanation:
Before doing anything, we need the speed in the same units as time:
V = 1.19m/s * 60s/min = 71.4m/min
Now, for part (a) we need position at t=1min and t=4.36min. Position at t=1min was 0m and at t=4.36min:
X=V*Δt = 71.4*(4.36-3.36)=71.4m
With this value, we can proceed to calculate average speed:
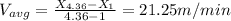
And for the acceleration:
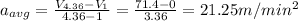
For part (c) and (d) we proceed similarly:
X=V*Δt = 71.4*(5.36-3.36)=142.8m
With this value, we can proceed to calculate average speed:
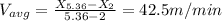
And for the acceleration: