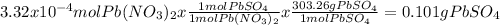Question

When aqueous solutions of K₂SO₄ and Pb(NO₃)₂ are combined, PbSO₄ precipitates. Calculate the mass, in grams, of the PbSO₄ produced when 1.66 mL of 0.2 M Pb(NO₃)₂ and 3.88 mL of 0.61 M K₂SO₄ are mixed. Calculate the mass to 3 significant figures.

Answer 1

0.101g PbSO₄

Step-by-step explanation:

To know an amount of a product we have to know first how many moles of reactants we have.

`1.66mLx0.2(molPb(NO_(3))_(2))/(L)x(1L)/(1000mL)= 3.32x10^(-4)mol Pb(NO_(3))_(2)`

`3.88mLx(0.61molK_(2)SO_(4))/(L)x(1L)/(1000mL) = 2.367x10^(-3)mol`
`K_(2)SO_(4)`

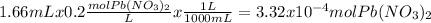
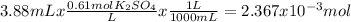
The reaction is:
`K_(2)SO_(4) + Pb(NO_(3))_(2)`⇒
`PbSO_(4) + 2KNO_(3)`

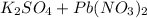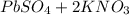
We can observe that per one mole of K₂SO₄ we need one mole of Pb(NO₃)₂, so Pb(NO₃)₂ is the limitant reactant and reaction will stop when it´s over.

`3.32x10^(-4)mol Pb(NO_(3))_(2)x(1molPbSO_(4))/(1molPb(NO_(3))_(2)) x(303.26gPbSO_(4))/(1molPbSO_(4))=0.101gPbSO_(4)`