Answer:
It will take
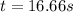 for the lead car to stop.
for the lead car to stop.
Step-by-step explanation:
Stage 1: The car moves at constant speed
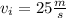 .
.
Stage 2: the car accelerates with
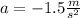 (the negative sign means it's decelerating with respect to the direction is moving).
(the negative sign means it's decelerating with respect to the direction is moving).
¿When will be the velocity be
 ?
?
From Kinematics:
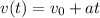
So, if final velocity is
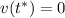
then,
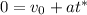 ⇒
⇒
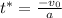 ⇒
⇒
 .
.