Question

The maintenance department at the main campus of a large state university receives daily requests to replace fluorecent lightbulbs. The distribution of the number of daily requests is bell-shaped and has a mean of 40 and a standard deviation of 4. Using the 68-95-99.7 rule, what is the approximate percentage of lightbulb replacement requests numbering between 40 and 48?

Answer 1

Answer: 47.5%

Explanation:

Given : The distribution of the number of daily requests is bell-shaped and has a mean of
`\mu=40` and a standard deviation of 40
`\sigma=4`.

To find : The approximate percentage of lightbulb replacement requests numbering between 40 and 48.

We can see that
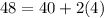`48=40+2(4)` i.e. 48 is two standard deviations ( to the right) from the mean. (1)

And 40 is the mean value (
`\mu`) itself . It means the lower limit lies at the center of the normal curve (at z=0).

It means we need only right the region which is 2 standard deviations from the mean (at z=2).

According to the 68-95-99.7 rule, 95% of the population falls within two deviation from the mean in both left and right side of the normal curve.

Then, the required area will be :
`(95\%)/(2)=47.5\%`

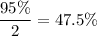
Hence, the approximate percentage of lightbulb replacement requests numbering between 40 and 48 = 47.5%