Question

A grocery supplier believes that the mean number of broken eggs per dozen is 0.8 with a standard deviation of 0.6. You buy 8 dozen eggs without checking them.

a) How many broken eggs do you expect to​ get?
b) What's the standard​ deviation?
c) Is it necessary to assume the cartons of eggs are​ independent? Why?

Answer 1

Explanation:

Given that a grocery supplier believes that the mean number of broken eggs per dozen is 0.8 with a standard deviation of 0.6.

Here we assume the cartons of eggs are independent

a) Mean value of eggs in 8 dozens = 8 Expected value in 1 dozen

`= 8(0,8) = 6.4`

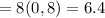
b) Var (8x) (Here 8 dozens)

`= 64 var(x)` (assuming independent)

Otherwise we have Var(x1+x2+x3+...)=sum of var of xis + cov (xi,xj)

Thus we have to assume independent to avoid covariance

So Var(8x)=64 Var(x)

STd dev for 8 dozens = 8(0,6) = 4.8

c) To avoid covariance i.e. variance among pairs of dozens we assume independence.