Answer:
The electric field at a distance r = 0.02 m is 14062.5 N/C.
Solution:
Refer to fig 1.
As per the question:
Radius of sphere, R = 0.04 m
Charge, Q =
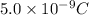
Distance from the center at which electric field is to be calculated, r = 0.02 m
Now,
According to Gauss' law:
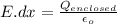
Now, the charge enclosed at a distance r is given by volume charge density:
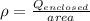
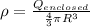
Also, the charge enclosed Q' at a distance r is given by volume charge density:
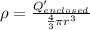
Since, the sphere is no-conducting, Volume charge density will be constant:
Thus
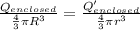
Thus charge enclosed at r:
![Q'_(enclosed) = \frac{Q_(enclosed)}{(r^(3))/(R^(3))]()
Now, By using Gauss' Law, Electric field at r is given by:
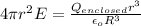
Thus
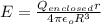
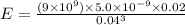
E = 14062.5 N/C