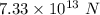Question

What are the (time varying) amplitudes of the E and H fields if summer sunlight has an intensity of 1150 W/m2 in any Town?

Calculate the relative strength of the gravitational and solar electromagnetic pressure forces of the sun on the earth.

Answer 1

The relative strength of the gravitational and solar electromagnetic pressure forces is
`7.33*10^(13)\ N`

Step-by-step explanation:

Given that,

Intensity = 1150 W/m²

(a). We need to calculate the magnetic field

Using formula of intensity

`I=(E^2)/(2\mu_(0)c)`

`E=\sqrt{2* I*\mu_(0)c}`

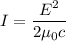
Put the value into the formula

`E=\sqrt{2*1150*4\pi*10^(-7)*3*10^(8)}`

`E=931.17\ N/C`

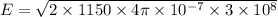
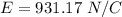
Using relation of magnetic field and electric field

`B=(E)/(c)`

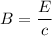
Put the value into the formula

`B=(931.17)/(3*10^(8))`

`B=0.0000031039\ T`

`B=3.10*10^(-6)\ T`

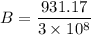
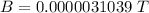
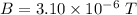
(2). The relative strength of the gravitational and solar electromagnetic pressure forces of the sun on the earth

We need to calculate the gravitational force

Using formula of gravitational

`F_(g)=(GmM)/(r^2)`

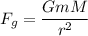
Where, m = mass of sun

m = mass of earth

r = distance

Put the value into the formula

`F_(g)=(6.67*10^(-11)*1.98*10^(30)*5.97*10^(24))/((1.496*10^(11))^2)`

`F_(g)=3.52*10^(22)\ N`

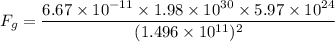
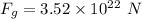
We need to calculate the radiation force

Using formula of radiation force

`F_(R)=(I)/(c)*\pi*R_(e)^2`

`F_(R)=(1150)/(3*10^(8))*\pi*(6.371*10^(6))^2`

`F_(R)=4.8*10^(8)\ N`

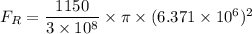
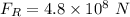
We need to calculate the pressure

`(F_(g))/(F_(R))=(3.52*10^(22))/(4.8*10^(8))`

`(F_(g))/(F_(R))=7.33*10^(13)\ N`

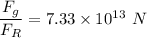
Hence, The relative strength of the gravitational and solar electromagnetic pressure forces is
`7.33*10^(13)\ N`