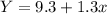Answer:
the equation of the least-squares line for the data is:
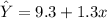
Explanation:
In a simple linear regression model, such as,
 , the coefficients bo and b1 are estimated through the method of least squares by the use of the equations:
, the coefficients bo and b1 are estimated through the method of least squares by the use of the equations:
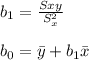
For the data provided you have to:
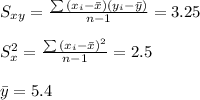 , thus:
, thus:
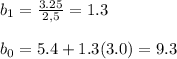
the equation of the least-squares line for the data is: