Answer:
18.86° , it will bend towards normal.
Step-by-step explanation:
For refraction,
Using Snell's law as:
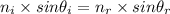
Where,
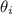 is the angle of incidence ( 25.0° )
is the angle of incidence ( 25.0° )
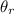 is the angle of refraction ( ? )
is the angle of refraction ( ? )
 is the refractive index of the refraction medium (n=1.7)
is the refractive index of the refraction medium (n=1.7)
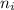 is the refractive index of the incidence medium ( n=1.3)
is the refractive index of the incidence medium ( n=1.3)
Hence,
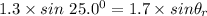
Angle of refraction =
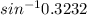 = 18.86°
= 18.86°
Since, the light ray is travelling from a material with a refractive index of 1.3 into a material with a refractive index of 1.7 or lighter to denser medium, it will bend towards normal.
The diagram is shown below: