Question

The electric field in a region is uniform (constant in space) and given by E-( 148.0 1 -110.03)N/C. An additional charge 10.4 nC (nano-coulombs) is placed into this region. What is the y component of the electric force on this charge?

Answer 1

`-1.144\ \mu C`

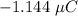
Step-by-step explanation:

Given:

• 
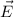  `\vec{E}` = uniform electric field in the space =
  `(148.0\ \hat{i}-110.0\ \hat{j})\ N/C`

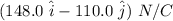
• Q = Charge placed in the region =
  `10.4 nC\ = 1.04* 10^(-8)\ nC`

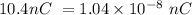
Assume:

• 
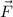  `\vec{F}` = Electric force on the charge due to electric field

We know that the electric field is the electric force applied on a unit positive charge i.e.,

`\vec{E}=\frac{\vec{F}}{Q}`

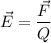
This means the electric force applied on this additional charge placed in the field is given by:

`\vec{F}=Q\vec{E}\\\Rightarrow \vec{F} =  1.04* 10^(-8)\ n C* (148.0\ \hat{i}-110.0\ \hat{j})\ N/C\\\Rightarrow \vec{F} = (1.539\ \hat{i}-1.144\ \hat{j})\ \mu N\\`

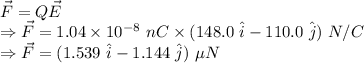
From the above expression of force, we have the following y-component of force on this additional charge.

`F_y = -1.144\ \mu N`

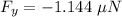
Hence, the y-component of the electric force on the this charge is
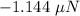`-1.144\ \mu N`.