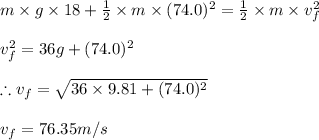Question

A cannon tilted up at a 33.0° angle fires a cannon ball at 74.0 m/s from atop a 18.0 m -high fortress wall. What is the ball's impact speed on the ground below?

Answer 1

The cannon ball hits the ground with a speed of 76.35 m/s.

Step-by-step explanation:

We shall use the conservation of energy principle to solve the problem

The initial energy of the cannon ball is the sum of the potential and the kinetic energies.

We know that potential energy =
`mgh`

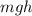
Applying the given values we obtain the initial potential energy as

`P.E=m* g* 18.0`

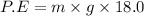
Similarly the initial kinetic energy of the ball equals

`K.E_(initial)=(1)/(2)mv_(o)^(2)`

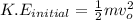
Applying values we get

`K.E_(initial)=(1)/(2)m(74.0)^(2)`

Thus the initial energy is thus
`E_(initial)=m* g* 18.0+(1)/(2)* m* (74.0)^(2)`

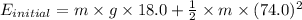
Now upon hitting the ground the only energy that the cannon ball will posses is kinetic energy since the potential energy of any object upon touching the surface of earth equals zero

Thus we have

`Energy_(final)=(1)/(2)mv_(f)^(2)`

Equating initial and final energies we get

`m* g* 18+(1)/(2)* m* (74.0)^(2)=(1)/(2)* m* v_(f)^(2)\\\\v_(f)^(2)=36g+(74.0)^(2)\\\\\therefore v_f=\sqrt{36* 9.81+(74.0)^(2)}\\\\v_f=76.35m/s`