Question

There are four candidates (A. B, C, and D) and 115 voters. When the points were tallied (using 4 points for frst, 3 points for second, 2 points for third, and 1 point for fourth) candidates. (Hint: Figure out how many points are packed in each ballot) ). A had 320 points, B had 330 points, and C had 190 points. Find how many points D had and give ranking of the Find the complete ranking of the candidates Choose the correct answer below

Answer 1

D had 310 points.

The final ranking is: B,A,D,C

Explanation:

The problems states that:

There are 115 votes.

Each ballot has a vote for first place, that counts 4 points, a vote for second place, that counts 3 points, a vote for third place, that counts 2 points and a vote for fourth place that counts 1 point. This means that each ballot has 4+3+2+1 = 10 points.

Since there are 115 voters, there are 115 ballots. This means that the total points of A,B,C and D combined must be equal to 115*10 = 1150. So:

`A + B + C + D = 1150`

We already know that:

A had 320 points, B had 330 points, and C had 190 points.

So:

`A + B + C + D = 1150`

`320 + 330 + 190 + D = 1150`

`D = 310`

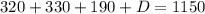
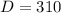
B had the most points, followed by A, D and C. So the final ranking is: B,A,D,C