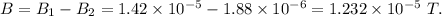Question

An extremely long wire laying parallel to the x -axis and passing through the origin carries a current of 250A running in the positive x -direction. Another extremely long wire laying parallel to the x -axis and passing through the y -axis at ry= 1.8m carries a current of 50A running in the negative x -direction. What is the magnitude of the net magnetic field that the wires generate on the y -axis at ry= −3.510m ?

Answer 1

`1.232* 10^(-5)\ T.`

Step-by-step explanation:

Given:

• Current through the wire, passing through the origin,
  `I_1 = 250\ A.`

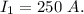
• Current through the wire, passing through the y axis,
  `r_y=1.8\ m.`,
  `I_2 = 50\ A.`

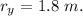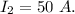
According to Ampere's circuital law, the line integral of magnetic field over a closed loop, called Amperian loop, is equal to
`\mu_o` times the net current threading the loop.

`\oint \vec B \cdot d\vec l=\mu_o I.`

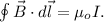
In case of a circular loop, the directions of magnetic field and the line element
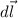`d\vec l`, both are along the tangent of the loop at that point, therefore,
`\vec B\cdot d\vec l = B\ dl`.

`\oint \vec B \cdot d\vec l = \oint B\ dl = B\oint dl.\\`

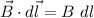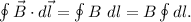
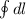`\oint dl` is the circumference of the Amperian loop =
`2\pi r`

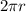
Therefore,

`B\ 2\pi r=\mu_o I\\B=(\mu_o I)/(2\pi r).`

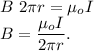
It is the magnetic field due to a current carrying wire at a distance r from it.

For the first wire, passing through the origin:

Consider an Amperian loop of radius 3.510 m, concentric with the axis of the wire, such that it passes through the point where magnetic field is to be found, therefore,
`r_1 = 3.510\ m.`

The magnetic field at the given point due to this wire is given by:

`B_1 = (\mu_o I_1)/(2\pi r_1)\\=(4\pi * 10^(-7)* 250)/(2\pi * 3.510)=1.42* 10^(-5)\ T.`

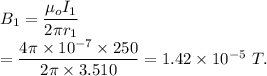
For the first wire, passing through the y-axis:

Consider an Amperian loop of radius (3.510+1.8) m = 5.310 m, concentric with the axis of the wire, such that it passes through the point where magnetic field is to be found, therefore,
`r_2 = 5.310\ m.`

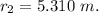
The magnetic field at the given point due to this wire is given by:

`B_2 = (\mu_o I_2)/(2\pi r_2)\\=(4\pi * 10^(-7)* 50)/(2\pi * 5.310)=1.88* 10^(-6)\ T.`

The directions of current in both the wires are opposite therefore, the directions of the magnetic field due to both the wires are also opposite to that of each other.

Thus, the net magnetic field at
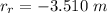`r_r=-3.510\ m` is given by

`B=B_1-B_2 = 1.42* 10^(-5)-1.88* 10^(-6)=1.232* 10^(-5)\ T.`