Answer:
Square of a rational number is a rational number.
Explanation:
Let m be a rational number. Thus, m can be written in the form of fraction
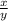 , where x and y are integers and
, where x and y are integers and
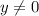 .
.
The square of m =
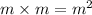
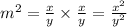
It is clearly seen, that
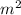 , can be easily written in the form of fraction and the denominator is not equal to zero.
, can be easily written in the form of fraction and the denominator is not equal to zero.
Hence,
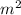 is a rational number.
is a rational number.
This can also be understood with the help of the fact that rational numbers are closed under multiplication that is product of a rational number is also a rational number.