Question

Find the error with this proof and explain how it mat be corrected in order to clearly prove the equation.

Prove that if m is an odd integer, then m2 is odd.

Proposed proof: Assume m is an odd integer. By definition of odd integer, m=2k+1, for some integer k.
This means that (2k + 1)^2 = 4k^2 + 1, so m is odd.

Answer 1

Explanation:

It is true that for any given odd integer, square of that integer will also be odd.

i.e if
`m` is and odd integer then
`m^(2)` is also odd.

In the given proof the expansion for
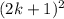`(2k + 1)^(2)` is incorrect.

By definition we know,

`(a+b)^(2) = a^(2) + b^(2) + 2ab`

∴
`(2k + 1)^(2) = (2k)^(2) + 1^(2) + 2(2k)(1)\\(2k + 1)^(2) = 4k^(2) + 1 + 4k`

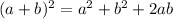
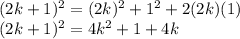
Now, we know
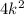`4k^(2)` and
`4k` will be even values

∴
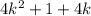`4k^(2) + 1 + 4k` will be odd

hence
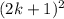`(2k + 1)^(2)` will be odd, which means
`m^(2)` will be odd.