Answer:
A ) displacement=75.69km
B) Angle
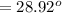
Step-by-step explanation:
This is a trigonometric problem:
in order to answer A and B , we first need to know the total displacement on east and north.
the tricky part is when the car goes to the direction northeast, but we know that:
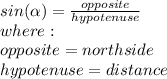
North'=9.86km
and we also know:
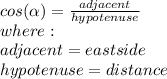
East'=18.54km
So know we have to total displacement
North=28km+North'=37.86km
East=50km+East'=68.54km
To calculate the total displacement, we have to find the hypotenuse, that is:
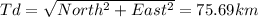
we can find the angle with:
