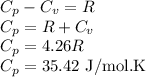Question

When 19.3 J was added as heat to a particular ideal gas, the volume of the gas changed from 56.7 cm^3 to 104 cm^3 while the pressure remained constant at 0.947 atm. (a) By how much did the internal energy of the gas change? If the quantity of gas present is 1.97 x 10^-3 mol, find the molar specific heat of the gas at (b) constant pressure and (c) constant volume.

Answer 1

a)
`C_p=35.42\ \rm J/mol.K`

b)
`C_v=27.1\ \rm J/mol.K`

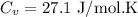
Step-by-step explanation:

Given:

• Heat given to the gas,
  `Q=19.3\ \rm J`
• Initial volume of the gas,
  `V_i=56.7\ \rm cm^3`

• Final volume of the gas,
  `V_f=104\ \rm cm^3`

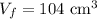
• Constant pressure of the gas,
  `P=0.947\ \rm atm`
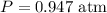
• Number of moles of the gas,
  `n=1.97*10^(-3)`

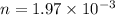
Let R be the gas constant which has value
`R=8.31432*10^3\ \rm N\ m\ kmol^(-1)K^(-1)`

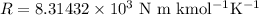
Work done in the process

`W=PdV\\W=0.947*(104-56.7)*10^(-3)\ \rm L\ atm\\W=44.8*10^(-3)*101.33\ \rm J\\W=4.43\ \rm J`

Now Using First Law of thermodynamics

`Q=W+\Delta U\\19.3=4.43+\Delta U\\\Delta U=14.77\ \rm J`

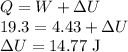
Let
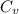`C_v` be the molar specific heat of the gas at constant volume given by

`\Delta U=nC_v\Delta T\\14.77=(C_v)/(R){PdV}\\14.77=(C_v)/(R)(0.987*10^5(104-56.7)*10^-6})\\C_v=3.26R\\C_v=27.1\ \rm J/mol.K`

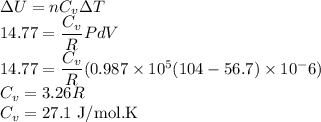
Also we know that

Let
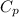`C_p` be the molar specific heat of the gas at constant pressure given by

`C_p-C_v=R\\C_p=R+C_v\\C_p=4.26R\\C_p=35.42\ \rm J/mol.K`