Question

Verify that the function(s) solve the following differential equations (DES): a) y' = -5y; y = 3e-5x b) y' = cos(3x); y = į sin(3x) + 7 c) y' = 2y; y = ce2x , where c is any real number. d) y" + y' – 6y = 0 ; yı = (2x, y2 = (–3x e) y" + 16y = 0; yı = cos(4x), y2 = sin(4x)

Answer 1

In the step-by-step explanation, the verifications are made.

Explanation:

a)
`y' = -5y`

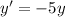
This one can be solved by the variable separation method

`y' = -5y`

`(dy)/(dx) = -5y`

`(dy)/(y) = -5dx`

`\int (dy)/(y)  = \int {-5} \, dx`

`ln y = -5x + C`

`e^(ln y) = e^(-5x + C)`

`y = Ce^(-5x)`

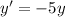
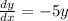
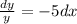
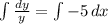
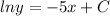
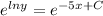
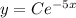
The value of C is the value of y when x = 0. If
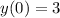`y(0) = 3`, then we have the following solution:

`y = 3e^(-5x)`

b)
`y' = cos(3x)`

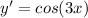
This one can also be solved by the variable separation method

`y' = cos(3x)`

`\int y' \,dy  = \int {cos(3x)} \, dx`

`y = (sin(3x))/(3) + K`

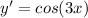
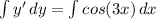
K is also the value of y, when x = 0. So, if
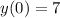`y(0) = 7`, we have the following solution.

`y = (sin(3x))/(3) + 7`

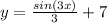
c)
`y' = 2y`

Another one that can be solved by the variable separation method

`y' = 2y`

`(dy)/(dx) = 2y`

`(dy)/(y) = 2dx`

`\int (dy)/(y)  = \int {2} \, dx`

`ln y = 2x + C`

`e^(ln y) = e^(2x + C)`

`y = Ce^(2x)`

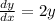
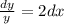
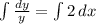
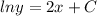
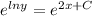
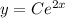
C is any real number depending on the initial conditions.

d)
`y'' + y' - 6y = 0`

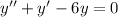
Here, the solution depends on the roots of the following equation:

`r^(2) + r - 6 = 0`

`r = (-1 \pm 5)/(2)`

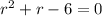
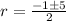
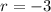`r = -3` or
`r = 2`.

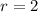So the solution is

`y(t) = c_(1)e^(-3t) + c2e^(2t)`

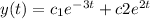
The values of
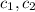`c_(1), c_(2)` depends on the initial conditions.

e)
`y'' + 16y = 0`

Again, we find the roots of the following equation:

`r^(2) + 16 = 0`

`r^(2) = -16`

`r = \pm 4i`

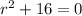
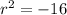
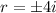
So we have the following solution

`y(t) = c_(1)cos(4t) + c_(2)sin(4t)`

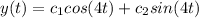
The values of
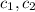`c_(1), c_(2)` depends on the initial conditions.