Answer:
4.3155 kW
Explanation:
Given,
speed of wind when enters into the turbine, V = 12 m/s
speed of wind when exits from the turbine, U = 9 m/s
mass flow rate of the wind = 137 kg/s
According to the law of conservation of energy
Energy generated = change in kinetic energy
Hence,energy generated in 1 sec can be given by
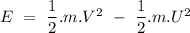
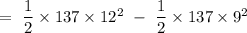

= 4315.5 J
So, the power generated in 1 sec will be given by
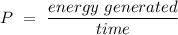
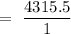
= 4315.5 W
= 4.13 kW
So, the power generated will be 4.13 kW.