Question

The monthly demand for a product is normally distributed with mean = 700 and standard deviation = 200.

1. What is probability demand will exceed 900 units in a month?

2. What is probability demand will be less than 392 units in a month?

Answer 1

Answer: a) 0.1587 b) 0.0618

Explanation:

Let x be the random variable that represents the monthly demand for a product.

Given : The monthly demand for a product is normally distributed with mean = 700 and standard deviation = 200.

i.e.
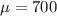`\mu=700` and
`\sigma=200`

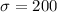
a) Using formula
`z=(x-\mu)/(\sigma)`, the z-value corresponds to x= 900 will be :

`z=(900-700)/(200)=1`

Now, by using the standard normal z-table , the probability demand will exceed 900 units in a month :-

`P(z>1)=1-P(z\leq1)=1-0.8413=0.1587`

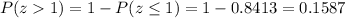
Hence, the probability demand will exceed 900 units in a month=0.1587

a) Using formula
`z=(x-\mu)/(\sigma)`, the z-value corresponds to x= 392 will be :

`z=( 392-700)/(200)=-1.54`

Now, by using the standard normal z-table , the probability demand will be less than 392 units in a month :-

`P(z<-1.54)=1-P(z<1.54)=1-0.9382=0.0618`

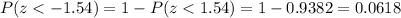
Hence, the probability demand will be less than 392 units in a month = 0.0618