Question

Computethe maximum height that a projectile can

reach if it is launchedwith speed V o at angle thetarelative to the horizontal. If an
object is thrown directly upwardswith a speed of 330m/s, the
typical speed of sound in the air atroom temperature, how high can
it get?

Answer 1

A.
`H=(v_(0)^(2)Sin^(2)\theta )/(2g)`

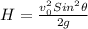
B. 5556.1 m

Step-by-step explanation:

A.

Launch speed, vo

Angle of projection = θ

The value of vertical component of velocity at maximum height is zero. Let the maximum height is H.

Use third equation of motion in vertical direction

`v_(y)^(2)=u_(y)^(2)+2a_(y)H`

`0^(2)=\left (v_(0)Sin\theta  \right )^(2)-2gH`

`H=(v_(0)^(2)Sin^(2)\theta )/(2g)`

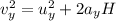
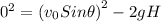
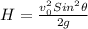
B.

u = 330 m/s

Let it goes upto height H.

V = 0 at maximum height

Use third equation of motion in vertical direction

`v^(2)=u^(2)+2as`

`0^(2)=330^(2)-2* 9.8* H`

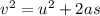
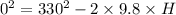
H = 5556.1 m