Question

You wish to prove that three propositions p1, p2, and p3 are equivalent. will it suffice to show that p1 --> p2, p2 --> p3, and p3 --> p1? justify your answer

Answer 1

It is sufficient to prove that
`p_1\implies p_2, p_2\implies p_3, p_3\implies p_1`

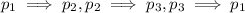
Explanation:

The propositions
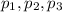`p_1,p_2,p_3` being equivalent means they should always have the same truth value. If one of them is true, then all of them must be true. And if one of them is false, then all of them must be false.

Suppose we've proven that
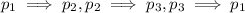`p_1\implies p_2, p_2\implies p_3, p_3\implies p_1` (call these first, second and third implications).

If
`p_1` was true, then by the first implication that we proved, it would follow that
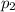`p_2` is also true. And then by the second implication that we prove it would follow then that
`p_3` is also true. Therefore the three of them would be true. Notice the reasoning would have been the same if we had started assuming that the one that was true was either
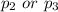`p_2~or~p_3`. So one of them being true makes all of them be true.

On the other hand, if
`p_1` was false, then by the third implication that we proved, it would follow that
`p_3` has to be false (otherwise
`p_1` would have to be true, which would be a contradiction). And then, since
`p_3` is false, by the second implication that we proved it would follow that
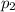`p_2` is false (otherwise
`p_3` would have to be true, which would be a contradiction). Therefore the three of them would be false. Notice the reasoning would have been the same if we had started assuming that the one that was false was either
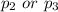`p_2~or~p_3`. So one of them being false makes all of them be false.

So, the three propositions always have the same truth value, and so they're all equivalent.